Question

Given a mean of 43.8 and a standard deviation of 8.7, within how many standard deviations of the mean do the values 60, 40, 35, 45, and 39 fall?

Answer 1

I think the answer is 0.

Answer 2

Answer with explanation:

Mean (
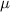`\mu`) = 43.8

Standard Deviation (
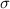`\sigma`) = 8.7

We can draw the normal curve and then show where these values lie.

Or we can use the equation,
`43.8 \pm 8.7 * k = \text{Value}`, use positive sign when ,number is greater than mean, and negative sign when number is smaller than mean, to find that , how many standard deviations of the mean do the values 60, 40, 35, 45, and 39 fall.

1.→ 43.8 +8.7 k= 60

8.7 k= 60 - 43.8

8.7 k= 16.2

`k=(16.2)/(8.7)\\\\k=1.8620\\\\k=1.86`

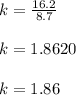
2.→ 43.8 - 8.7 k=40

43.8-40 = 8.7 k

8.7 k= 3.8

`k=(3.8)/(8.7)\\\\k=0.4367\\\\k=0.44`

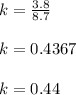
3.→43.8 - 8.7 k=35

43.8-35 = 8.7 k

8.7 k= 8.8

`k=(8.8)/(8.7)\\\\k=1.011\\\\k=1.01`

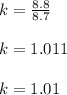
4.→43.8 + 8.7 k=45

43.8-45 = -8.7 k

-8.7 k= -1.2

8.7 k= 1.2

`k=(1.2)/(8.7)\\\\k=0.1379\\\\k=0.14`

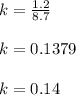
5.→43.8 -8.7 k=39

43.8-39 = 8.7 k

8.7 k= 4.8

`k=(4.8)/(8.7)\\\\k=0.5517\\\\k=0.55`