Question

Derivative by first principle logsecx²​

Answer 1

`\displaystyle (d)/(dx)[\log \big( \sec (x^2) \big)] = (2x \tan x^2)/(\ln 10)`

General Formulas and Concepts:

Calculus

Differentiation

• Derivatives
• Derivative Notation

Basic Power Rule:

1. f(x) = cxⁿ
2. f’(x) = c·nxⁿ⁻¹

Derivative Rule [Chain Rule]:
`\displaystyle (d)/(dx)[f(g(x))] =f'(g(x)) \cdot g'(x)`

Explanation:

Step 1: Define

Identify

`\displaystyle y = \log \big( \sec (x^2) \big)`

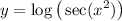
Step 2: Differentiate

1. Logarithmic Differentiation [Derivative Rule - Chain Rule]:
   `\displaystyle y' = ((\sec x^2)')/(\ln (10) \sec x^2)`
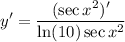
2. Trigonometric Differentiation [Derivative Rule - Chain Rule]:
   `\displaystyle y' = (\sec x^2 \tan x^2 (x^2)')/(\ln (10) \sec x^2)`
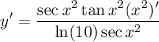
3. Simplify:
   `\displaystyle y' = (\tan x^2 (x^2)')/(\ln 10)`
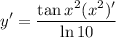
4. Basic Power Rule:
   `\displaystyle y' = (2x \tan x^2)/(\ln 10)`

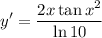
Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Differentiation