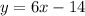Question

(01.05) what is the slope-intercept form equation of the line that passes through (3, 4) and (5, 16)? (1 point) y = 6x − 14 y = −6x + 14 y = −6x − 14 y = 6x + 14

Answer 1

(3,4)(5,16)
slope = (16 - 4) / (5 - 3) = 12/2 = 6

y = mx + b
slope(m) = 6
use either of ur points (3,4)...x = 3 and y = 4
sub and find b, the y int
4 = 6(3) + b
4 = 18 + b
4 - 18 = b
-14 = b

so ur equation is : y = 6x - 14

Answer 2

The answer is
`y=6x-14`

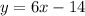
Explanation:

In order to determine the slope-intercept form equation, we need to know the formula. The formula needs a slope (m) and a point (x,y) that belongs to the equation.

`y-y_1=m(x-x_1)\\m=slope\\(x_1,y_1)=point`

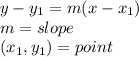
For m:

`m=(y_2-y_1)/(x_2-x_1)`

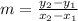
So, first we have to define every variable and then we replace them into the formula:

`P_1=(3,4)\\P_2=(5,16)\\m=(16-4)/(5-3)\\m=(12)/(2)=6`

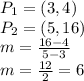
It doesn't matter the order of how you define the points. The result will be the same.

Then:

`y-4=6*(x-3)\\y=6*(x-3)+4\\y=6x-18+4\\y=6x-14`

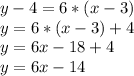
Finally, the slope-intercept form equation is
`y=6x-14`