Question

Suppose a line has slope 4 and passes through the point (-2, 5). Which other point must also be on the graph?

A) (2, 6) B) (-1, 9) C) (-1, 1) D) (-6, 4)

Answer 1

`\bf slope = {{ m}}= \cfrac{rise}{run}\implies 4\implies \cfrac{4}{1}`

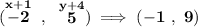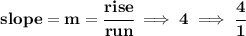
so the rise is 4 units and the run is 1 unit, ok.

now, from point -2, 5, if we move 4/1, that is, "1 unit over the x-axis, and 4 units over the y-axis", we'll find another point, now, a fraction can be positive so long both top and bottom are the same sign, so -4/-1 is the same as 4/1 and therefore, if we move +4 and +1 over the axes we get a point, or if we move -4 and -1 we also get another point, and any other subsequent points from there on.

so, hmm let's check
`\bf (\stackrel{x+1}{-2}~,~\stackrel{y+4}{5})\implies (-1~,~9)`

Answer 2

D) -6,4. because if it has a slope of 4 which is positive it has to have an uphill slope which passes through -2,5, in order for that to happen you plot it and make the line and count the rise over run till you have the slope of 4.