Question

Which interval contains a local minimum for the graphed function?

A.) [-4, -2.5]
B.) [-2, -1]
C.) [1, 2]
D.) [2.5, 4]

Answer 1

The correct option is D. The interval [2.5, 4] contains a local minimum for the graphed function

Explanation:

According to the definition of local mimima, a function has local minima at c if

`f(c)<f(x)`

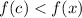
for all values of x. where,
`x\in [c-\epsilon, c+\epsilon]`

From the graph it is clear that the given function has local minima at (-0.44,-4.3) and (3,-4).

`-0.44\\otin [-4, -2.5], 3\\otin [-4, -2.5]`

Therefore option A is incorrect.

`-0.44\\otin [-2, -1], 3)\\otin [-2, -1]`

Therefore option B is incorrect.

`-0.44\\otin [1, 2], 3\\otin [1, 2]`

Therefore option C is incorrect.

`-0.44\\otin [2.5, 4], 3\in [2.5, 4]`

Therefore option D is correct.

Answer 2

Answer : Option D is correct i.e [2.5,4]

Explanation :

Suppose our function is f(x)

then the value of f(x) is minimum where

it reaches -0.44 and 3 with two different intervals .

As we know that for finding the local minimum ,

the criteria is that f'(x)=0 .

So, here

f'(-0.44)=0 and

f'(3)=0

both are the local minimum point for the function f(x)

but -0.44 is the global minimum point .

In our case for [2.5,4] is the required interval where f(x) reaches its local minimum.