Question

Which functions are symmetric with respect to the y-axis? Check all that apply.

f(x) = |x|

f(x) = |x| + 3

f(x) = |x + 3|

f(x) = |x| + 6

f(x) = |x – 6|

f(x) = |x + 3| – 6

Answer 1

f(x)=/x/ I believe that its my right answer

Answer 2

The correct options are 1, 2 and 4.

Explanation:

The vertex form of an absolute function is

`f(x)=|x-a|+b`

Where, (a,b) is the vertex of the function and x=a is axis of symmetry.

The function is symmetric with respect to the y-axis. It means the axis of symmetry is x=0. It is possible if the value of a in the vertex form is 0.

In option 1,

`f(x)=|x|`

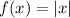
Here, a=0 and b=0.

Since the value of a=0, therefore it is symmetric with respect to the y-axis.

In option 2,

`f(x)=|x|+3`

Here, a=0 and b=3.

Since the value of a=0, therefore it is symmetric with respect to the y-axis.

In option 3,

`f(x)=|x+3|`

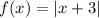
Here, a=-3 and b=0.

Since the value of a≠0, therefore it is not symmetric with respect to the y-axis.

In option 4,

`f(x)=|x|+6`

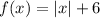
Here, a=0 and b=6.

Since the value of a=0, therefore it is symmetric with respect to the y-axis.

In option 5,

`f(x)=|x-6|`

Here, a=6 and b=0.

Since the value of a≠0, therefore it is not symmetric with respect to the y-axis.

In option 6,

`f(x)=|x+3|-6`

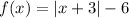
Here, a=-3 and b=-6.

Since the value of a≠0, therefore it is not symmetric with respect to the y-axis.

Hence the correct options are 1, 2 and 4.