Question

A freight train slows down as it approaches a train yard. If a force of -3.8 x 10^6 is required to provide an acceleration of -0.33 m/s^2, what is the train's mass?

Answer 1

The train's mass is approximately 1.15 x
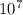`10^7` kg.

According to Newton's second law of motion, the force acting on an object is equal to the mass of the object multiplied by its acceleration (F = ma).

We are given the force (-3.8 x
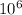`10^6` N) and the acceleration (-0.33 m/
`s^2`), and we need to find the mass (m).

Rearranging the equation, we get:

m = F / a

Plugging in the values:

m = (-3.8 x
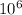`10^6` N) / (-0.33 m/
`s^2`)

m ≈ 1.15 x
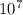`10^7` kg