Question

What are the explicit equation and domain for a geometric sequence with a first term of 4 and a second term of -8?

Answer 1

I believe the answer is;

an = 4(−2)n − 1; all integers where n ≥ 1

Answer 2

The explicit equation for the given geometric sequence is
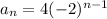`a_n=4(-2)^(n-1)`. The domain for the geometric sequence is all positive integers except 0.

Explanation:

It is given that the first term of the geometric sequence is 4 and the second term is -8.

`a_1=4,a_2=-8`

The common ratio for the sequence is

`r=(a_2)/(a_1)=(-8)/(4)=-2`

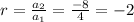
The explicit equation for a given geometric sequence is

`a_n=ar^(n-1)`

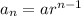
where, a is first term, n is number of term and r is common ratio.

The explicit equation for the given geometric sequence is

`a_n=4(-2)^(n-1)`

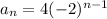
Here n is the number of term. So, the value of n is must be a positive integer except 0.

Therefore the domain for the geometric sequence is all positive integers except 0.