Question

Which of the following is always irrational?

A. the sum of two fractions
B. the product of a fraction and a repeating decimal
C. the sum of a terminating decimal and the square root of a perfect square
D. the product of a repeating decimal and the square root of a non-perfect square

Answer 1

Correct choice is D

Explanation:

Option A. The sum of two fractions is a fraction, a fraction is always rational number.

Option B. The product of a fraction and a repeating decimal can be rational number. For example,

`(3)/(2)\cdot 0.\overline{3}=(3)/(2)\cdot (1)/(3)=(1)/(2).`

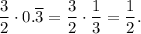
Option C. The sum of a terminating decimal and the square root of a perfect square is always rational number, because the terminating decimal is a decimal that ends and the square root of a perfect square is a natural number. The product of decimal that ends and natural number is rational number.

Option D. The product of a repeating decimal and the square root of a non-perfect square is always irrational, because the square root of a non-perfect square is irrational number and when multiplying irrational number by fraction (any repeating decimal can be written as fraction) you get irrational number.