Answer: The required value of the first term is 8.
Step-by-step explanation: We are given to find the first term of the arithmetic sequence for which the third term is 126 and sixty fourth term is 3725.
We know that
the nth term of an arithmetic sequence with first term a and common difference d is given by
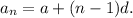
According to the given information, we have

and

Subtracting equation (i) from equation (ii), we get

From equation (i), we get
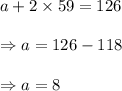
Thus, the required value of the first term is 8.