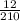Answer:
Explanation:
Strawberry cakes = 2
Pineapple cakes = 6
Chocolate cakes = 7
Total cakes = 15
Now we are given that Vincent selects a cake randomly without looking, and he will give a pineapple cake to the first child
So, probability of getting a pine apple cake in first draw =
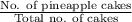
=
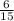
Now total no. of remaining cakes = 15-1 =14
Now we are given that Vincent selects a cake randomly without looking, and he will give a strawberry cake to the second child
So, So, probability of getting a strawberry cake in second draw=
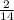
So, the probability that he will give a pineapple cake to the first child and then a strawberry cake to the second child =

=
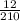
Hence Option A is true.
The probability that he will give a pineapple cake to the first child and then a strawberry cake to the second child is