Question

Select the correct inequality for the graph below: A solid line passing through points (1, 2) and (2, 5) has shading below. y < 3x − 1 y ≤ 3x − 1 y ≥ 3x − 1 y > 3x − 1

Answer 1

Here is your answer:

Solving the equation:

• 
  `(5-2)/(2-1)= 3`
• 
  `(y - y1)/((x - x1) )`
• 
  `y-5=3(x-2)`
• 
  `y= 3x- 6+ 5`
• "
  `y= 3x-1` " or option B.

Hope this helps!

Answer 2

Step 1

Find the equation of the line that passes through points
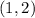`(1, 2)` and
`(2, 5)`

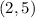
Find the slope of the line

The formula to calculate the slope is equal to

`m=(y2-y1)/(x2-x1)`

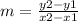
substitute the values

`m=(5-2)/(2-1)`

`m=(3)/(1)`

`m=3`

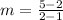
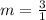
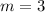
Find the equation of the line

The equation of the line into slope-point form is equal to

`y-y1=m(x-x1)`

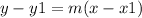
we have

`m=3`

`(1, 2)`

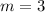
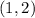
substitutes

`y-2=3(x-1)`

`y=3x-3+2`

`y=3x-1`

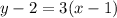
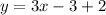
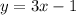
Step 2

Find the equation of the inequality

we know that

The solution is the shaded area below the solid line

therefore

the inequality is

`y\leq 3x-1`

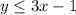
the answer is

`y\leq 3x-1`

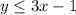
see the attached figure to better understand the problem