Question

Determine the solution set of (x - 4)^2 = 12.

{4 + 2√3, 4 - 2√3}
{4 - 2√3, 4 - 2√3}
{2√3 + 4, 2√3 - 4}

Answer 1

x = 4 + 2 sqrt(3) or x = 4 - 2 sqrt(3) thus {4 + 2√3, 4 - 2√3} is your answer!

Explanation:

Solve for x over the real numbers:

(x - 4)^2 = 12

Take the square root of both sides:

x - 4 = 2 sqrt(3) or x - 4 = -2 sqrt(3)

Add 4 to both sides:

x = 4 + 2 sqrt(3) or x - 4 = -2 sqrt(3)

Add 4 to both sides:

Answer: x = 4 + 2 sqrt(3) or x = 4 - 2 sqrt(3)

Answer 2

Expand (x - 4)^2:

`(x - 4) \cdot (x - 4) = (x \cdot x) + (x \cdot -4) + (-4 \cdot x) + (-4 \cdot -4)`

`x^2 - 4x - 4x + 16 = \boxed{x^2 - 8x + 16 = 12}`

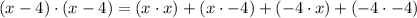
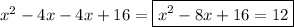
Subtract 12 from both sides to get one side to equal 0:

`x^2 - 8x + 4 = 0`

Find the values of a, b, and c in this quadratic equation:

`x^2 \ | \ a = 1`

`-8x \ | \ b = -8`

`4 \ | \ c = 4`

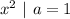
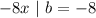
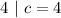
The quadratic formula is expressed as follows:

`\begin{array}{*{20}c} {x = \frac{{ - b \pm \sqrt {b^2 - 4ac} }}{{2a}}} & {{\rm{when}}} & {ax^2 + bx + c = 0} \\ \end{array}`

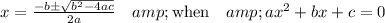
Plug in our values into the formula:

`\begin{array}{*{20}c} {x = \frac{{ 8 \pm \sqrt {(-8)^2 - 4(1)(4)} }}{{2(1)}}} \end{array}`

`\begin{array}{*{20}c} {x = \frac{{ 8 \pm \sqrt {64 - 16} }}{{2}}} \end{array}`

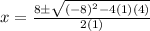
Simplify the square root:

`√(64 - 16) = √(48)`

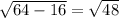
Prime factorize the square root:

`√(48) = √(4 \cdot 12) = √(2 \cdot 2 \cdot 3 \cdot 4) = √(2 \cdot 2 \cdot 2 \cdot 2 \cdot 3)`

Take any number that is repeated twice in the square root, and move it outside:

`√(2 \cdot 2) = 2`

`√((2 \cdot 2) \cdot (2 \cdot 2) \cdot 3) = 2 \cdot 2 √(3) = \boxed{4 √(3)}`

`\begin{array}{*{20}c} {x = \frac{{ 8 \pm 4 √(3) }}{{2}}} \end{array}`

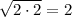
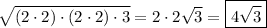
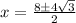
Solve the plus and minus:

`(8 + 4 √(3))/(2) = \boxed{4 + 2√(3)}`

`(8 - 4 √(3))/(2) = \boxed{4 - 2√(3)}`

`\boxed{x = 4 + 2√(3) \ \& \ 4 - 2√(3)}`

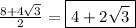
The answer is {4 + 2√3, 4 - 2√3}.