The phase shift of function g(x) is 5/2, which indicates that the graph of this sine function is shifted 2.5 units to the right.
Step-by-step explanation:
The phase shift of function
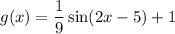 can be found by analyzing the argument of the sine function. The general form for a sine function with a phase shift is f(x) = sin(bx - c), where c/b is the phase shift. Looking at the given function, we have a multiplier of 2 before the x and a subtraction of 5. Thus the phase shift is 5/2, because we divide the subtracted number by the multiplier of x.
can be found by analyzing the argument of the sine function. The general form for a sine function with a phase shift is f(x) = sin(bx - c), where c/b is the phase shift. Looking at the given function, we have a multiplier of 2 before the x and a subtraction of 5. Thus the phase shift is 5/2, because we divide the subtracted number by the multiplier of x.
In this specific case, the phase shift is positive, meaning the graph of the sine function has shifted to the right. A positive phase shift always indicates a shift to the right and vice versa for a negative phase shift.