Answer:

Explanation:
We have been given an image of a right triangle and we are asked to find the measure of angle A of our given triangle.
We can see that hypotenuse and opposite side to angle A is given. Since sine represents the relation between opposite and hypotenuse of a right triangle, so we will use sine to find the measure of angle A.
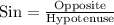
Upon substituting our given values in above relation we will get,
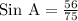
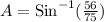
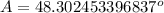
Upon rounding our answer to nearest degree we will get,

Therefore, the measure of angle A to the nearest degree is 48 degrees.