From the diagram you can see that the graph of function has three x-intercepts. If you need the coordinates of these points, then you should substitute y=0 and solve the received equation:
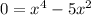 .
.
First, factor this expression:
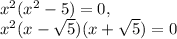 .
.
This means that
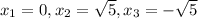 .
.
The x-intercepts are points
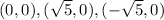 .
.
Answer: 3 x-intercepts.