Question

Given that sec theta= -37/12, what is the value of cot theta , for pie/2 < theta< pie?

A.-35/12
B.-12/35
C.12/35
D.35/12

Answer 1

The answer is B. Thank me in the future!

always remember ~Positive Vibes~

Answer 2

Option B is correct.

Explanation:

Given that

`\sec{\theta}=-(37)/(12)`

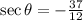
and the terminal point
`\theta` is in quadrant 2,i.e
`(\pi)/(2)<\theta<\pi`

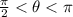
we have to find the value of
`\cot \theta`

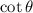
As in second quadrant
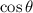`cos(\theta)` is negative.

`cos(\theta)=\frac{1}{\sec{\theta}}=(1)/((-37)/(12))=-(12)/(37)`

As in second quadrant all trigonometric functions are negative except
`sin(\theta) \thinspace and\thinspace \csc{\theta}`

`sin{\theta}=\pm\sqrt{1-\cos^2{\theta}}=\pm\sqrt{1-(-(12)/(37))^2)}=\pm\sqrt{1-(144)/(1369)}=\pm\sqrt{(1225)/(1369)}=(35)/(37)`

`\cot{\theta}=(cos(\theta))/(sin(\theta))=(-(12)/(37))/((35)/(37))=-(12)/(35)`

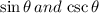
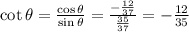
Option B is correct.