Question

Match each trigonometric function with its Unit Circle definition. Note that Angle A is correctly oriented for the Unit Circle definition, its terminal side intersects the Unit Circle at the point (x, y), and neither x nor y is equal to zero.

1. y
2. x
3. y/x
4. 1/y
5. 1/x
6. x/y

a. sin A
b. csc A
c. tan A
d. sec A
e. cos A
f. cot A

Answer 1

The first diagram below shows a circle with a radius of 1 (unit circle). The circle is drawn on a Cartesian graph with (0,0) as the center of the circle.

From the second diagram, we can determine the value of sin(Θ) = y
and cos(Θ) = x

We can further deduce that
tan(Θ) =
`(y)/(x)`
sec(Θ) =
`(1)/(cos(Θ))` =
`(1)/(x)`
cosec(Θ) =
`(1)/(sin(Θ))` =
`(1)/(y)`
cot(Θ) =
`(cos(Θ))/(sin(Θ))` =
`(x)/(y)`

Answer 2

The required matching is a-1, b-4, c-3, d-5, e-2, f-6.

Explanation:

Unit Circle is circle having radius 1 units and centered at origin.

The terminal side intersects the Unit Circle at the point (x, y), and neither x nor y is equal to zero.

So, perpendicular of triangle is y, base is x and hypotenuse is 1 unit.

It a right angled triangle,

`\sin A=(perpendicular)/(hypotenuse)\Rightarrow (y)/(1)=y`

`\csc A=(1)/(\sin A)=(1)/(y)`

`\tan A=(perpendicular)/(base)\Rightarrow (y)/(x)`

`\sec A=(hypotenuse)/(base)=(1)/(x)`

`\cos A=(base)/(hypotenuse)=(x)/(1)=x`

`\cot A=(1)/(\tan A)=(x)/(y)`

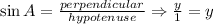
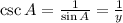
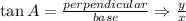
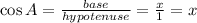
Therefore the required matching is a-1, b-4, c-3, d-5, e-2, f-6.