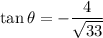Answer:
The value of tan θ is:
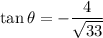
Explanation:
From the given right angled triangle we have:
Base of the triangle i.e. OA is: √33 units
and Perpendicular length of triangle i.e. AB is: 4 units
Hence, in the given right angled triangle we will use the trignometric ratio corresponding to the angle (360°-θ) as:

As we know that:
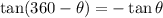
Hence,
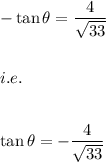
Hence, the answer is: