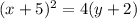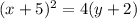
the equation for a parabola with focus (h, k+p) and directrix y=k-p is
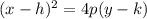
so using your directrix y=-3, and knowing directrix is y=k-p, you have
-3=k-p.
similarly, knowing the focus is defined by (h,k+p) and your focus is (-5,-1)
you have the equation -1=k+p.
you now have a system of equations
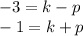
which you can solve using any method, I will use elimination.
adding down
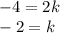
you now have k and can find p using either equation.
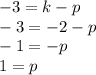
.
now you plug those in, getting the answer