Answer:
The tide is 12 feet height at t=2.
Explanation:
Given:
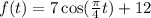
It is cosine function which represents the tide of light sea.
It has maximum of 19 feet at t=0
It has minimum of 5 feet at t=4
The sea repeats cycle every 8 hours. (Period = 8)
We need to find height of tide after 2 hours.
We will put t=2 into equation and solve for f(t)
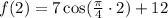
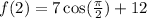
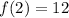
Please see the attachment for graphical result.
Hence, The tide is 12 feet height at t=2.