Question

Amir pitches a baseball at an initial height of 6 feet, with a velocity of 73 feet per second. If the batter misses, about how long does it take the ball hit the ground?

Hint: Use H(t) = -16t^2+vt+s

Answer 1

Hey! The correct time is 4.64 seconds.

Answer 2

4.643 seconds

Explanation:

We have been given that

s = 6 feet

v = 73 feet per second

Substituting these values in the formula
`H(t)=-16t^2+vt+s`

`H(t)=-16t^2+73t+6`

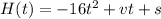
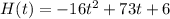
When the ball hits the ground, the height becomes zero. Thus, H(t)=0

`-16t^2+73t+6=0`

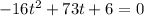
We solve the equation using quadratic formula
`x_(1,\:2)=(-b\pm √(b^2-4ac))/(2a)`

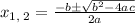
Substituting the values
`a=-16,\:b=73,\:c=6`

`t_(1,\:2)=(-73\pm √(73^2-4\left(-16\right)6))/(2\left(-16\right))\\\\=(-73\pm√(5713))/(-2\cdot \:16)\\\\=-0.081,4.643`

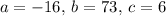
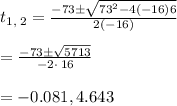
time can't be negative. Hence, t = 4.643.

Hence, the ball will take 4.643 seconds to hit the ground.