Answer:
The graph is symmetric about the x-axis.
Explanation:
- The graph is symmetric about the polar axis(x-axis) if we replace (r,θ) with (r,-θ) then it is equivalent to the original equation.
- The graph is symmetric about the y-axis if we replace (r,θ) with (-r,-θ) then it is equivalent to the original equation.
- The graph is symmetric about the pole(origin) if we replace (r,θ) with (-r,θ) then it is equivalent to the original equation.
Here we have equation as:

- when we replace (r,θ) with (r,-θ) we have:
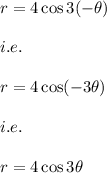
( Since, we know that:
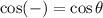 )
)
Hence, the graph is symmetric about the x-axis.
- when we replace (r,θ) with (-r,-θ)
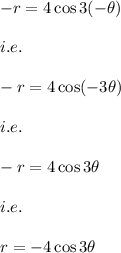
Hence, we observe that on replacing the function the two graphs are not equivalent.
Hence, the graph is not symmetric about the y-axis.
- when we replace (r,θ) with (-r,θ) we have:
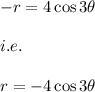
Hence, we observe that on replacing the function the two graphs are not equivalent.
Hence, the graph is not symmetric about the pole(origin)