Question

Which are true if figure DEFG is reflected across the x-axis? Check all that apply.

1.D(0, 4) → D'(0, –4)
2.E(–2, 0) → E'(–2, 0)
3.The perpendicular distance from G' to the x-axis will equal 2 units.
4.The perpendicular distance from D' to the x-axis will equal 8 units.
5.The orientation will be preserved.

Answer 1

1st, 2nd, and 3rd are correct:)

Have an awesome day!

Answer 2

Answer: The correct options are 1,2 and 3.

Step-by-step explanation:

If a figure reflected across the x-axis then the x-coordinate remains same but the sign of y-coordinate changes.

According to the reflection rule across the x-axis,

`(x,y)\rightarrow(x,-y)`

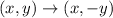
From the given figure it is noticed that the coordinate of point D(0,4) and E(-2,0).

After reflection,

`D(0,4)\rightarrow D'(0,-4)`

`E(-2,0)\rightarrow E'(-2,0)`

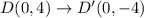
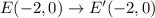
Therefore the option 1 and 2 are correct.

From the given figure it is noticed the distance of point G from the x-axis is 2, therefore the distance from the G' to x-axis is also 2, because the distance of preimage and image are equal from the line of reflection.

Therefore, the option 3 is correct.

From the given figure it is noticed the distance of point D from the x-axis is 4, therefore the distance from the D' to x-axis is also 4.

Therefore, the option 4 is incorrect.

From the below figure it is clearly noticed that the orientation will not be preserved. Because the sides are not equal, so the reflection will change the orientation.