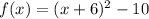Answer:
The quadratic function in vertex form is
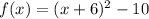
Explanation:
Given equation of quadratic function
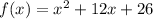
We have to write the given quadratic function in vertex form.
For a given quadratic function
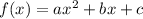 can rewritten in standard form by completing the square.
can rewritten in standard form by completing the square.
The standard form of quadratic function
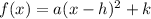 , where (h,k) denotes the vertex of the equation.
, where (h,k) denotes the vertex of the equation.
If a is positive, the graph opens upward, and if a is negative, then it opens downward.
Consider the given function
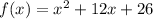
Using algebraic identity
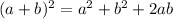
We have a = x and 2ab = 12x ⇒ b = 6
Adding
 term to complete square and subtract so that the function remain same, we have,
term to complete square and subtract so that the function remain same, we have,
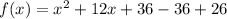
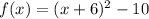
Which is in form of standard form of quadratic function.
Thus, The quadratic function in vertex form is