Question

the height of a triangle is 7 cm longer than its base. The area of the triangle is 60 cm². What is the base of the triangle?

Answer 1

base is 8cm, and height is 15 cm

Answer 2

the base of the triangle is 8 cm

Explanation:

Area of a triangle(A) is given by:

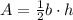`A =(1)/(2)b \cdot h` ....[1]

where b is the base and h is the height of the triangle respectively.

As per the statement:

the height of a triangle is 7 cm longer than its base.

⇒
`h = b+7`

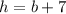
It is also given that: The area of the triangle is 60 cm²

⇒
`A = 60` cm²

Substitute the given values in [1] we have;

`60 = (1)/(2)b \cdot(b+7)`

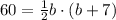
Multiply both sides by 2 we have;

`120 = b(b+7)`

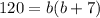
or

`b^2+7b =120`

⇒
`b^2+7b-120=0`

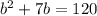
Now factorize this equations:

`b^2+15b-8b-120=0`

⇒
`b(b+15)-8(b+15)=0`

⇒
`(b-8)(b+15)=0`

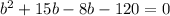
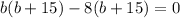
By zero product property we have;

b-8 = 0 and b+15 = 0

⇒b = 8 and b = -15

Since, the base of the triangle cannot be in negative.

⇒b = 8 cm

Therefore, the base of the triangle is 8 cm