Question

A 50.0-g Super Ball traveling at 29.5 m/s bounces off a brick wall and rebounds at 20.0 m/s. A high-speed camera records this event. If the ball is in contact with the wall for 4.00 ms, what is the magnitude of the average acceleration of the ball during this time interval

Answer 1

The magnitude of the average acceleration of the ball during this time interval is 1.238 x 10⁴ m/s².

Step-by-step explanation:

Given;

mass of the super ball, m = 50 g = 0.05 kg

initial velocity of the ball, u = 29.5 m/s

final velocity of the ball, v = -20.0 m/s (negative because it rebounds)

time of contact of the ball and the wall, t = 4 ms = 4 x 10⁻³ s

The force exerted on the brick wall by the ball is given as;

`F = ma\\\\ma = (m(v-u))/(t) \\\\a = (v-u)/(t) \\\\a = ((-20) - 29.5)/(4.0 \ * \ 10^(-3)) \\\\a = (-49.5)/(4.0 \ * \ 10^(-3)) \\\\a = -1.238 * 10^4 \ m/s^2\\\\|a| = 1.238 * 10^4 \ m/s^2`

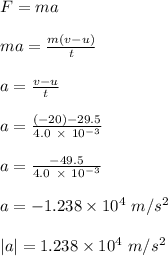
Therefore, the magnitude of the average acceleration of the ball during this time interval is 1.238 x 10⁴ m/s².