Question

Eighty-two percent of employees make judgements about their co-workers based on the cleanliness of their desk. You randomly select 7 employees and ask them if they judge co-workers based on this criterion. The random variable is the number of employees who judge their co-workers by cleanliness. Which outcomes of this binomial distribution would be considered unusual

Answer 1

0,1, 2 and 3 employees judging their co-workers by cleanliness would be considered unusual.

Explanation:

Binomial probability distribution

Probability of exactly x sucesses on n repeated trials, with p probability.

Can be approximated to a normal distribution, using the expected value and the standard deviation.

The expected value of the binomial distribution is:

`E(X) = np`

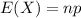
The standard deviation of the binomial distribution is:

`√(V(X)) = √(np(1-p))`

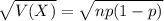
An outcome is considered unusual if it is more than 2.5 standard deviations from the mean.

Eighty-two percent of employees make judgements about their co-workers based on the cleanliness of their desk.

This means that
`p = 0.82`

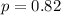
You randomly select 7 employees and ask them if they judge co-workers based on this criterion.

This means that
`n = 7`

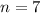
Mean:

`E(X) = np = 7*0.82 = 5.74`

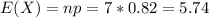
Standard deviation:

`√(V(X)) = √(np(1-p)) = √(7*0.82*0.18) = 1.02`

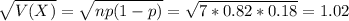
Two and a half standard deviations above the mean:

Will be higher than 7, so no possible values.

Two and a half standard deviations below the mean:

`5.74 - 2.5*1.02 = 3.19`

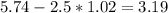
So 0,1, 2 and 3 employees judging their co-workers by cleanliness would be considered unusual.