Question

Explain how the angle-angle-side congruence theorem is an extension of the angle-side-angle congruence theorem. be sure to discuss the information you would need for each theorem.

Answer 1

The interior angle measures of a triangle add up to 180 degrees. Thus, if you are given angle-angle-side, you can solve for the third angle measure and essentially have angle-side-angle because the given side will now be the included side.

Explanation:

Answer 2

ASA congruence theorem: If two angles and the included side of one triangle are equal to the corresponding angles and side of another triangle, the triangles are congruent.

AAS congruence theorem: If two angles and the non-included side of one triangle are equal to the corresponding angles and side of another triangle, the triangles are congruent.

Consider two triangles ΔABC and ΔA'B'C' . If AB=A'B', m∠A=m∠A' and m∠B=m∠B', then two triangles ΔABC and ΔA'B'C' are congruent by ASA theorem.
Now find ∠C and ∠C':

`m\angle C=180^(\circ)-m\angle A-m\angle B` and
`m\angle C'=180^(\circ)-m\angle A'-m\angle B'=180^(\circ)-m\angle A-m\angle B=m\angle C`.
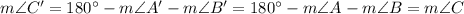
You have AB=A'B', m∠A=m∠A' , m∠C=m∠C' and that is the condition of AAS congruence theorem. These thoughts show you that AAS theorem is straight extension of ASA theorem.