Question

The value of Sara's new car decreases at a rate of 8% each year

1. write an exponential function to model the decrease in the car's value each month.
2. write an exponential function to model the decrease in the car's value each week.
3. write an exponential function to model the decrease in the car's value each day.
4. what relationship is there between the amount of decrease and the time interval measured

Answer 1

A=p(1-0.08/12)^12t

A=p(1-0.08/52)^52t

A=p(1-0.08/360)^360t

0.08÷12=0.0067
0.08÷52=0.0015
0.08÷360=0.00022

Answer 2

Since, the exponential decay function that models the present value,

`f(x)=a(1-r)^x`

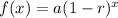
Where, a shows the initial value,

r shows rate of decay per period,

x is the number of periods,

Given,

Annual rate = 8 % = 0.08

1. 1 year = 12 months

So, monthly rate, r =
`(0.08)/(12)`

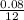
Number of periods in x years, t = 12x

Thus, the function would be,

`f(x)=a(1-(0.08)/(12))^(12x)`

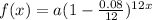
2. 1 year = 52 weeks

So, weekly rate, r =
`(0.008)/(52)=(1)/(6500)`

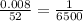
Number of periods in x years, t = 52x

Thus, the function would be,

`f(x)=a(1-(0.08)/(52))^(52x)`

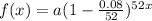
3. 1 year = 365 days

So, daily rate, r =
`(0.008)/(365)=(1)/(45625)`

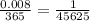
Number of periods in x years, t = 365x

Thus, the function would be,

`f(x)=a(1-(0.08)/(365))^(365x)`

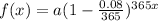
4. Since, with increasing time the value of car will decrease,

Hence, there is an inverse relation between the amount of decrease and the time interval measured.