Question

The equation T^2=A^3 shows the relationship between a planet’s orbital period, T, and the planet’s mean distance from the sun, A, in astronomical units, AU. If planet Y is twice the mean distance from the sun as planet X, by what factor is the orbital period increased?

Answer 1

From a series of derivations in the equation, I got C. 2^2/3 though I'm not very sure with that. Hope that helps.

Hugs **

Answer 2

`2\sqrt2` or 2.828

Explanation:

The given equation is

`T^2=A^3`

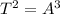
Let the mean distance from the sun of planet X is A then

mean distance from the sun of planet Y is 2A.

Therefore, we have

For planet X-

`T_x^2=A^3....(i)`

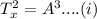
For planet Y-

`T_y^2=(2A)^3\\\\T_y^2=8A^3....(ii)`

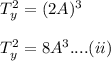
Divide equation (i) and (ii)

`(T_x^2)/(T_y^2)=(A^3)/(8A^3)\\\\(T_x^2)/(T_y^2)=(1)/(8)\\\\(T_x)/(T_y)=(1)/(2\sqrt2)\\\\T_y=2\sqrt2T_x`

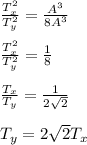
Therefore, we can conclude that orbital period is increase by a factor of
`2\sqrt2` or 2.828