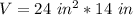Question

What is the volume of the triangular prism
Hight 10in
Base 14 in
Length 6

Answer 1

`V=336\ in^3`

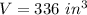
Explanation:

Triangular Prism

Given a triangular prism of base area A and height H, its volume is obtained with the formula:

V = AH

The area of the base is calculated as follows:

`A = (WL)/(2)`

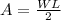
Where W is the width and L is the length. Both dimensions must be perpendicular.

The triangle of the base has a hypotenuse equal to 10 in and the width is 6 in, the length is calculated by:

`L=√(10^2-6^2)`

`L=√(100-36)=√(64)`

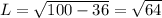
L = 8 in

The area is:

`A = (6*8)/(2)`

`A = 24\ in^2`

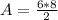
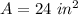
Finally, the volume is:

`V = 24\ in^2*14\ in`

`\boxed{V=336\ in^3}`