Answer:
The height of the maple is 98.6 feet
Explanation:
Similar Triangles
The triangles formed by each tree's height, their shadow, and the projection of their shadow in the ground are right and similar triangles since the sun has the same elevation at the same time in the same place. It's shown in the image attached.
Curtis sees a maple tree of unknown height Hm and a pine tree of height Hp=20.68 feet. He also measures the projection of the shadow of the pine of Xp=4.2 feet and the same measure of the maple of Xm=20.02 feet.
Since both triangles are similar, the proportion between their sides is equal, thus:

Solving for Hm:
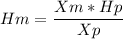
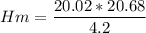
Hm = 98.6 feet
The height of the maple is 98.6 feet