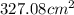Answer:
Explanation:
The area of a circular sector is calculated with this expression:
 ; where
; where
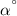 the central angle, and
the central angle, and
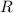 is the radius.
is the radius.
Then, we replace all values and solve for A:
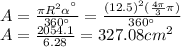
In the problem, we used
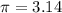 , and
, and
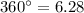 , because the problem is asking to use radians, and we cannot operate radians with grades, it would be wrong.
, because the problem is asking to use radians, and we cannot operate radians with grades, it would be wrong.
Therefore, the answer is