Question

The ratio of the surface area of two cubes is 49:81. Find the ratio of there volumes.​

Answer 1

343 : 729

Explanation:

If the ratio of the sides of a cube = a : b , then

ratio of areas = a² : b² and

ratio of volumes = a³ : b³

Given ratio of areas = 49 : 81, then

ratio of sides =
`√(49)` :
`√(81)` = 7 : 9 , so

ratio of volumes = 7³ : 9³ = 343 : 729

Answer 2

343 : 729

Explanation:

A cube is made up of 6 square faces. If each face has a side length of a, we can find the surface area of that cube with the formula
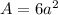`A=6a^2`, since we'll have 6 faces with area a². Let's call the edge length of the first cube a and the edge length of the second cube b. The ratio between their surface areas is then 6a² : 6b², or simply a² : b². If we compare this to our given ratio 49 : 81, we can see that a² = 49 and b² = 81, or, square rooting both equations, a = 7 and b = 9.

The volume of a cube with a side length a is a³, so the ratio between our cubes here must be a³ : b³. Using the values for a and b we just found, this ratio becomes 7³ : 9³, which we can simplify to 343 : 729.