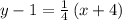Question

Write an equation in point-slope form of the line that passes through (-4,1) and (4,3).

Answer 1

An equation in point-slope form of the line that passes through (-4,1) and (4,3) will be:

`y-1=(1)/(4)\left(x+4\right)`

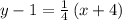
Explanation:

Given the points

• (-4,1)

• (4,3)

Finding the slope between the points (-4,1) and (4,3)

`\mathrm{Slope}=(y_2-y_1)/(x_2-x_1)`

`\left(x_1,\:y_1\right)=\left(-4,\:1\right),\:\left(x_2,\:y_2\right)=\left(4,\:3\right)`

`m=(3-1)/(4-\left(-4\right))`

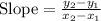
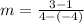
Refine

`m=(1)/(4)`

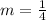
Point slope form:

`y-y_1=m\left(x-x_1\right)`

where

• m is the slope of the line

• (x₁, y₁) is the point

in our case,

• m = 1/4

• (x₁, y₁) = (-4,1)

substituting the values m = 1/4 and the point (-4,1) in the point slope form of line equation.

`y-y_1=m\left(x-x_1\right)`

`y-1=(1)/(4)\left(x-\left(-4\right)\right)`

`y-1=(1)/(4)\left(x+4\right)`

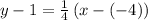
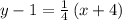
Thus, an equation in point-slope form of the line that passes through (-4,1) and (4,3) will be:

`y-1=(1)/(4)\left(x+4\right)`