Question

Suppose that the functions u and w are defined as follows :

u(x)= x^2+6

w(x)= \sqrt{x+9}

Find the following:
(u o w) (7) =
(w o u) 7) =

Answer 1

(u o w) (7) = 22

(w o u) 7) = 8

Explanation:

We are given:

`u(x)= x^2+6\\w(x)= √(x+9)`

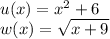
We need to find:

a) (u o w) (7)

First we will find (u o w) (x) and then we will find (u o w) (7)

We know that (u o w) (x) = u(w(x))

Put value of w(x) into u(x)

we have:

`u(x)=x^2+6\\Put\: x =√(x+9)\\u(w(x))=(√(x+9))^2+6\\u(w(x))=x+9+6\\ u(w(x))=x+15`

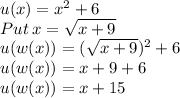
Now finding (u o w) (7)

We know that: (u o w) (7) = u(w(7))

`u(w(x))=x+15\\Put\:x=7\\u(w(7))=7+15\\u(w(7))=22`

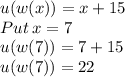
So, (u o w) (7) = 22

b) (w o u) (7)

First we will find (w o u) (x) and then we will find (w o u) (7)

We know that (w o u) (x) = w(u(x))

Put value of u(x) into w(x)

we have:

`w(x)= √(x+9)\\Put\:x=x^2+6\\w(u(x))= √((x^2+6)+9)\\w(u(x))= √(x^2+6+9)\\w(u(x))= √(x^2+15)`

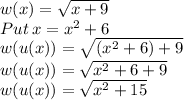
Now finding (w o u) (7)

We know that (w o u) (7) = w(u(7))

`w(u(x))= √(x^2+15)\\Put\:x=7\\w(u(7))= √((7)^2+15)\\w(u(7))= √(49+15)\\w(u(7))= √(64)\\w(u(7))= 8`

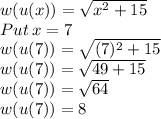
So, (w o u) (7) = 8