Question

7.   Find the range of the function f(x) = 4x – 1 for the domain {–1, 0, 1, 2, 3}.  (1 point)

{–5, –3, 0, 7, 11}

{–5, –4, –3, –2, –1}

{–11, –7, –3, 1, 5}

{–5, –1, 3, 7, 11}



8.   Find the slope of the graph of the following: 9x – 3y = 15  (1 point)

–3

3

–1/3

1/3





9.   How many solutions does this system have?
x – 2y = 2
        y = –2x + 5  (1 point)

infinitely many solutions

no solutions

two solutions

one solution



10.   Solve the system by either substitution or elimination.

3x – 5y = 21
2x + y = 1  (1 point)

(2, –3)

(2, 3)

(–2, –1)

(–2, 1)



11.   What is the slope-intercept form of the equation 4x + 2y = 6?  (1 point)

y = 2x + 3

y = 2x – 3

y = –2x – 3

y = –2x + 3

Answer 1

7. {-5, -1, 3, 7, 11}
8. 3
9. one solution
10. (2, -3)
11. 7=2x+3

Answer 2

Ques 7)

{–5, –1, 3, 7, 11}

Ques 8)

3

Ques 9)

one solution.

Ques 10)

(2,-3)

Ques 11)

y= -2x+3

Explanation:

Ques 7)

We have to find the range of the function:

f(x)=4x-1.

Given that domain is:

{-1,0,1,2,3}

The range of the function is the value of the function corresponding to the points of the domain.

so when x= -1

f(x)=4×(-1)-1=-4-1=-5

when x=0

f(x)=4×0-1=0-1=-1

when x=1

f(x)=4×1-1=4-1=3

when x=2

f(x)=4×2-1=8-1=7

when x=3

f(x)=4×3-1=12-1=11

Hence, the range of the function is:

{-5,-1,3,7,11}

Ques 8)

We have to find the slope of the graph of the equation:

`9x-3y=15`

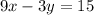
Firstly we will write our equation in the slope intercept form as:

`y=mx+c`

Where m is the slope of the graph and c is the y-intercept of the graph.

Hence,

`9x-15=3y\\\\3y=9x-15\\\\y=(9x-15)/(3)\\\\y=(9)/(3)x-(15)/(3)\\\\y=3x-5`

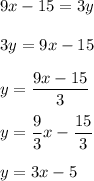
Hence, the slope of the equation is:

3

Ques 9)

We have to find the number of solution of the system of equations as:

x – 2y = 2

y = –2x + 5

On solving the system of equation by substitution method:

we put second equation in first to obtain:

x-2(-2x=5)=2

x+4x-10=2

5x=2+10

5x=12

x=12/5=2.4

Also putting the value of x in second equation we obtain:

y=0.2

Hence, we obtain a unique value of x and y on solving these system of equations.

So it has only one solution.

Ques 10)

We are given system of equation as:

3x-5y=21

2x+y=1

the second equation could also be written as:

y=1-2x-----------(a)

on putting the value of y in first equation we get:

3x-5(1-2x)=21

3x-5+10x=21

13x-5=21

13x=21+5

13x=26

x=26/13=2

i.e. x=2

on putting the value of x in equation (a) we get:

y=1-2×(2)=1-4=-3

i.e. y= -3

Hence the solution is:

(2,-3)

Ques 11)

We have to convert the equation:

4x+2y=6 into slope-intercept form of the line.

We know that the slope intercept form of the line is given as:

y=mx+c

where m is the slope of the line and c is the y-intercept.

2y=-4x+6

on dividing both side of the equation by 2 we obtain:

y=-2x+3