Answer: The correct option is (B) -172.
Step-by-step explanation: We are given to find the 32nd term of the arithmetic sequence where the 1st term and 13th term are
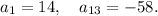
We know that
the n-th term of an arithmetic sequence with first term
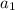 and common difference
and common difference
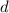 is given by
is given by
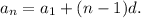
According to the given information, we have
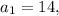
and

Therefore, the 32nd term will be
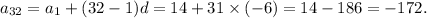
Thus, the 32nd term is -172.
Option (B) is correct.