Question

The width of a rectangle is 7 inches less than its length. the area of the rectangle is 120 square inches. solve for the dimensions of the rectangle.

Answer 1

I hope this helps you




width=length-7


Area=width×length


120=(length-7).length


15.8=(length -7).length


length =15


width =15-7=8

Answer 2

Answer: The required dimensions of the rectangle are 15 inches and 8 inches.

Step-by-step explanation: Given that the The width of a rectangle is 7 inches less than its length and the area of the rectangle is 120 square inches.

We are to find the dimensions of the rectangle.

Let, 'l' and 'b' represents the length and breadth of the rectangle.

So, b = l - 7.

Now, the AREA of the rectangle will be

`A=l* b\\\\\Rightarrow 120=l* (l-7)\\\\\Rightarrow 120=l^2-7l\\\\\Rightarrow l^2-7l-120=0\\\\\Rightarrow l^2-15l+8l-120=0\\\\\Rightarrow l(l-15)+8(l-15)=0\\\\\Rightarrow (l-15)(l+8)=0\\\\\Rightarrow l-15=0,~~~~~l+8=0\\\\\Rightarrow l=15,~~~~~~~\Rightarrow l=-8.`

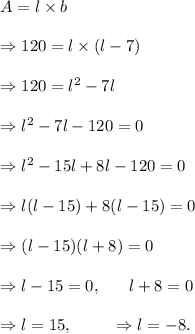
Since the length cannot be negative, so l = 15 inches.

Therefore, the breadth of the rectangle will be (15 - 8) = 7 inches.

Thus, the required dimensions of the rectangle are 15 inches and 8 inches.