Answer:
Option A is correct.
Explanation:
Given an isosceles trapezoid has base angles of 45° and bases of lengths 8 and 14. we have to find the area of isosceles trapezoid.
An isosceles trapezoid has base angles of 45° and bases of lengths 8 and 14.
From the figure attached , we can see an isosceles trapezoid ABCD,
AB = 8cm and CD=14cm
So we have to find the value of AE which is the height of Trapezoid in order to find area.
In ΔAED
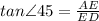
⇒
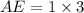
∴ AE = DE =3cm
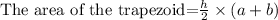
h=3cm, a=14cm, b=8cm
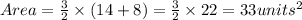
hence,
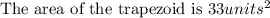
Option A is correct.