Answer:
Scale Factor ≈ 0.67
P'Q' Length = 10
Explanation:
To find the scale factor, a relationship must be found between the original points, and the dilated points. To do this, simply divide the new points by the old points.
P to P' :
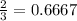 AND
AND

Q to Q' :
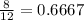 AND
AND

Therefore, the scale factor is 0.6667 ≈ 0.67
The length of P'Q' can be calculated with the distance formula:

Alternatively, you could multiply the length of PQ with the scale factor to find P'Q':
15*0.6667=10