Question

 A flying cannonball’s height is described by formula y=−16t2+300t. Find the highest point of its trajectory. In how many seconds after the shot will cannonball be at the highest point?

Answer 1

the trajectory is 1.5497476 NE It will be at its highest point approximately 7.89 seconds after being shot Hope this helps :)

Answer 2

The cannonball will be at the highest point in 9.375 seconds after the shot.

Explanation:

Suppose we have a quadratic function in the format:

`y(t) = at^(2) + bt + c`

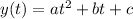
The maximum point will happen when:

`t_(v) = -(b)/(2a)`

The point will be:

`y_(MAX) = y(t_(v))`

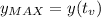
In this problem:

`y = -16t^(2) + 300t`

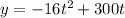
So
`a = -16, b = 300`

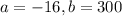
We have to find
`t_(v)`

`t_(v) = -(300)/(2(-16)) = 9.375`

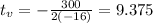
The cannonball will be at the highest point in 9.375 seconds after the shot.