Answer:
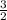 will be the slope of the line perpendicular to xy.
will be the slope of the line perpendicular to xy.
Explanation:
The equation of line xy is ( y-3 ) =
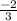 (x-4)
(x-4)
y =
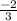 ( x-4 ) + 3
( x-4 ) + 3
y =
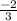 x +
x +
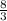 + 3
+ 3
y =
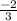 x +
x +

So the line has slope =
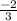 and y-intercept =
and y-intercept =
 .
.
Let m is the slope of a line perpendicular to line xy.
We know that for perpendicular lines having slopes m₁ and m₂
m₁ × m₂ = -1
Therefore, for m₁ = m and m₂ =
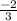
mx (
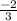 ) = -1
) = -1
m = (
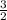 )
)
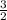 will be the slope of the line perpendicular to xy.
will be the slope of the line perpendicular to xy.