Hello!
The figure is made up of a cylinder and a hemisphere. To the nearest whole number, what is the approximate volume of this figure? Use 3.14 to approximate π
Data: (Cylinder)
h (height) = 7 in
r (radius) = 2.5 in (The diameter is 5 being twice the radius)
Adopting:
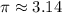
V (volume) = ?
Solving: (Cylinder volume)

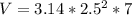
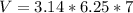

Note: Now, let's find the volume of a hemisphere.
Data: (hemisphere volume)
V (volume) = ?
r (radius) = 2.5 in (The diameter is 5 being twice the radius)
Adopting:
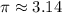
If: We know that the volume of a sphere is
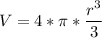 , but we have a hemisphere, so the formula will be half the volume of the hemisphere
, but we have a hemisphere, so the formula will be half the volume of the hemisphere
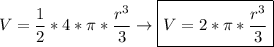
Formula: (Volume of the hemisphere)
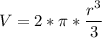
Solving:
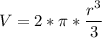
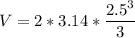
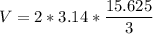
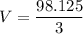

Now, to find the total volume of the figure, add the values: (cylinder volume + hemisphere volume)
Volume of the figure = cylinder volume + hemisphere volume
Volume of the figure = 137.38 in³ + 32.70 in³

_______________________
I Hope this helps, greetings ... Dexteright02! =)