Answer:
Use an equation to determine whether f(x) or g(x) is the correct model. Create an equation for the radius of the flask in terms of its volume.
Because the height is the same as the radius, write this formula for the volume of the flask:
V=

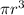
Rewrite the formula, solving for r:
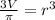
![\sqrt[3]{(3V)/(\pi ) } =r](https://img.qammunity.org/2022/formulas/mathematics/high-school/2lodkwmr0n223r63n86e4o0bldpuqy4b8i.png)
Now, graph the function and try to identify the values that are in the table.
The graph of the function does match the table of values. So, the table for f(x) can be used to find the radius of the flask. Notice that the graph for g(x) doesn’t match the graph of the formula, so exclude it as the answer.
Explanation:
This is the sample answer for edmentum/plato so you should change it a bit.