Answer and Explanation:
Given : A store sells different kinds of candy at $1, $1.50, $2, and $3 per kilogram.
To find : How many kilograms of each kind of candy does $3 buy?
Explain why the price of 1 kg and the amount of candy that $3 can buy are inversely proportional quantities?
Solution :
The total amount spent on buying candies is $3.
A store sells different kinds of candy at $1, $1.50, $2, and $3 per kilogram.
When the cost of candies is $1 per kg.
Amount of candies bought is
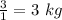
When the cost of candies is $1.50 per kg.
Amount of candies bought is
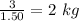
When the cost of candies is $2 per kg.
Amount of candies bought is
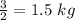
When the cost of candies is $3 per kg.
Amount of candies bought is
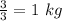
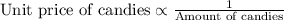
The amount spent on candies is constant.
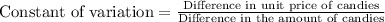
The difference in the unit price of candies = 3-2=1
The difference in the amount of candies = 1-1.5=-0.5
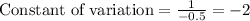
The negative sign indicates the inverse proportionality.