Question

Solve for x and check for extraneous roots:
`( (8)/( x^(2) -1))+( (4)/(x+1)) = 1`

Answer 1

Right away you know that you can't have
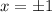
`x=\pm1` as possible solutions because either of these make the first term's denominator 0, while
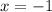
`x=-1` also makes the second term's denominator 0.

With this in mind, let's fix
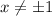
`x\\eq\pm1`, which allows you to write


`\frac8{x^2-1}+\frac4{x+1}=1\iff8+4(x-1)=x^2-1`

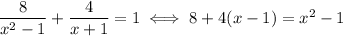
Moving everything to one side, you have


`x^2-4x-5=0\iff (x-5)(x+1)=0\implies x=5\text{ or }x=-1`

We omitted
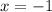
`x=-1` from the solution set, so this is an extraneous root and we're left with a solution of
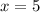
`x=5`.