Answer
Explanation :
It is given that:
mass of baseball,
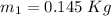
mass of canoe,

velocity of baseball,
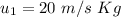
velocity of canoe,

Let v is the velocity of the first canoe moving after the pitch.
Now, using conservation of momentum as
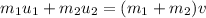
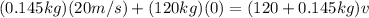
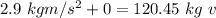
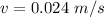
Hence, it is the required solution.