Question

A car moving at a speed of 36 km/h reaches the foot of a smooth

incline, when the engine is switched off. If the angle of inclination is 30

degrees, how much distance does the automobile travel up the ramp?

Answer 1

d = 10.2 m

Step-by-step explanation:

When the car travels up the inclined plane, its kinetic energy will be used to do the work in climbing up. So according to the law of conservation of energy, we can write that:

`Kinetic\ Energy\ of\ the \ Car = Work\ Done\ while\ moving\ up\ the\ plane\\(1)/(2)mv^(2) = Fd`

where,

m = mass of car

v = speed of car at the start of plane = (36 km/h)(1000 m/1 km)(1 h/3600 s)

v = 10 m/s

F = force on the car in direction of inclination = W Sin θ

W = weight of car = mg

θ = Angle of inclinition = 30°

d = distance covered up the ramp = ?

Therefore,

`(1)/(2)mv^(2) = mgdSin\theta\\(1)/(2)v^(2) = gdSin\theta\\(1)/(2)(10\ m/s)^(2) = d(9.81\ m/s^(2)) Sin\ 30^(0)`

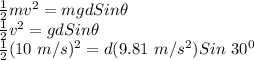
d = 10.2 m